Abstract : High-performance lead-free dielectric ceramics with simultaneously high energy storage density and power density are in high demanded for pulse power systems. To realize excellent energy-storage characteristics, a strategy to enhance antiferroelectricity and construct a local random field simultaneously was proposed in this study. Based on the above strategy, a series of (1-x)NaNbO3-xBi(Ni1/2Sn1/2)O3 [xBNS, x = 0.05, 0.10, 0.15, 0.20, and 0.22] solid solutions were designed and fabricated. An ultrahigh energy storage density (Utotal) of 7.35 J/cm³, and recoverable energy density (Urec) of 5.00 J/cm³ were achieved in the 0.10BNS ceramics. In addition, an adequate stability of energy storage properties at a range of temperatures (20–140 °C), frequencies (1–100 Hz), and fatigue test durations (1–104 cycles) were realized in 0.10BNS ceramics. 0.10BNS ceramics displayed a high current density of 1005 A/cm², an ultrahigh power density of 100.5 MW/cm³, and an ultrashort discharge time of 46.5 ns? This remarkable performance not only justified our strategy but also confirmed 0.10BNS ceramics as a promising candidate for energy storage.
Keywords : Lead-free ceramicsEnergy storageNaNbO3-BasedPower density
1. Introduction
In recent years, owing to the increasing demand for clean and renewable energy storage materials, the search for high energy storage density and power density (PD) materials has become an important research direction in the development of efficient and compact energy storage devices [[1], [2], [3]]. Dielectric capacitors, as one of the three representative energy storage methods, are the primary choice due to their high PD, high working voltage and adequate thermal endurance [[4], [5], [6], [7]]. The recoverable energy storage density (Urec) and energy storage efficiency (η) of ceramic dielectric capacitors can be calculated from polarization-electric field (P-E) loops by applying the following equations [8,9]:

where Pmax represents the maximum polarization, Pr the remnant polarization, E the electric field, and Utotal the total energy storage density. A strong breakdown electric field (Eb) strength, high Pmax, and near-zero Pr result in excellent energy storage performance (ESP).
Unlike other ceramics containing dielectric capacitors [linear dielectric (LD) and ferroelectric (FE)], antiferroelectric (AFE) ceramics usually present a high Pmax, low Pr and strong Eb [[10], [11], [12], [13]]. Pb(Zr, Ti)O3 (PZT)-based ceramics, as representative AFE ceramics, have been extensively studied in the energy storage field due to their high dielectric permittivity (ε) and strong polarization [14]. However, alternatives to lead-based materials are inevitably being sought due to their structural defects and environmental impact [2,15]. Among the alternative lead-free antiferroelectric materials, AgNbO3 single bond (AN) and NaNbO3 (NN)-based ceramic systems were considered as potential energy storage materials. A series of chemical modifications further increased the Urec values of AN-based ceramics to a range of 2–4.5 J/cm³ [11,16,17]. Nevertheless, due to the presence of the precious metal Ag and a difficult fabrication process [18], further applications of the ceramics in the energy storage field are severely limited.
Among AFE materials, NN ceramics are the most likely to be commercialized, owing to their high dielectric permittivity (high Pmax value), wide band gap (high Eb value), the absence of potassium (leading to facile preparation) and low bulk density (facilitating miniaturization) [19,20]. The crystal structure of NN ceramics at room temperatures (RT) was identified as the AFE orthogonal P phase with a Pbma space group [21]. However, through a series of hysteresis loop tests, it was determined that NN ceramics exhibited weak ferroelectricity at RT. This may have been caused by the similarity between the free energy in the AFE orthogonal P phase and that in the FE orthogonal Q phase [21,22]. Hence, it is necessary to reduce the quantity of the FE phase to improve ESP. Methods such as stabilizing the AFE phase and introducing relaxor characteristics, have been proposed to reduce FE in lead-free dielectric ceramics. For example, Qi et al. obtained an ultrahigh Urec of 12.2 J/cm³ in a 0.76NN-0.24(Bi0.5Na0.5)TiO3 relaxor antiferroelectric ceramic [23]. Qu et al. simultaneously realized a high Urec of 2.31 J/cm³ and η of 80.2% in NN-based ceramics via constructing the coexistence of nanodomains and polar nano-regions (PNRs) [24]. Through inducing antiferroelectricity and constructing a local random field simultaneously, Ye et al. synthesized 0.90NN-0.10Bi(Mg2/3Nb1/3)O3 ceramics with a large Urec of 2.8 J/cm³ [19]. Of particular significance is a study reporting the combination of antiferroelectric and relaxor characteristics in 0.55(Na0.5Bi0.5)TiO3-0.45(Sr0.7Bi0.2)TiO3 multilayer ceramic capacitors, Li et al. realized excellent ESP with both a high Urec of 9.5 J/cm³ and a large η of 90% [25]. Recently, Yang et al. summarized various modifications for improving the energy storage properties of NN and predicted the challenges and opportunities that further development of lead-free anti-ferroelectrics would encounter [12]. Although a significant amount of work has been done, there are still few researches reporting that an ultrahigh Urec (>4.5 J/cm³) and a superior power density (>100 MW/cm³) can be achieved simultaneously in NN-based or other lead-free bulk ceramics.
In previous studies, BNS was used as an effective dopant to significantly enhance the energy storage of BaTiO3-based and Bi0.5Na0.5TiO3-based ceramics [26,27]. However, there is no equivalent study for NN-based ceramic systems. To address the above problems, we propose the addition of a perovskite BNS to improve the ESP of NN ceramics. The improvements implemented in this study are illustrated in Fig. 1 and are described below. First, the AFE phase was stabilized by reducing the tolerance factor [28,29]. The introduction of A-site ions with smaller ionic radii, such as Bi3+ (1.17 Å, CN = 12), and B-site ions with larger ionic radii, such as (Ni0.5Sn0.5)3+ [R(Ni0.5Sn0.5)3+ = 0.69 Å, CN = 6], promoted antiferroelectricity, thus enhancing energy storage density. Second, a local random field was formed by introducing non-isovalent ions to A-sites and/or B-sites [19]. The incorporation of non-isovalent ions (Bi3+, Ni2+, and Sn4+) to the A- and B-sites caused a mismatch strain and a local charge imbalance, leading to a local random field, which disrupted the long-range ferroelectric order and decreased Pr. Third, Eb strength was enhanced by decreasing grain size (Eb ∝ G-a, G and a are average grain size, and a constant, respectively). Finally, the hybridization of Bi 6s and O 2p orbitals was used to enhance the polarity of the A-sites to compensate for the introduction low polarity ions (Ni2+, Sn4+) at B-sites, giving rise to high Pmax [30].
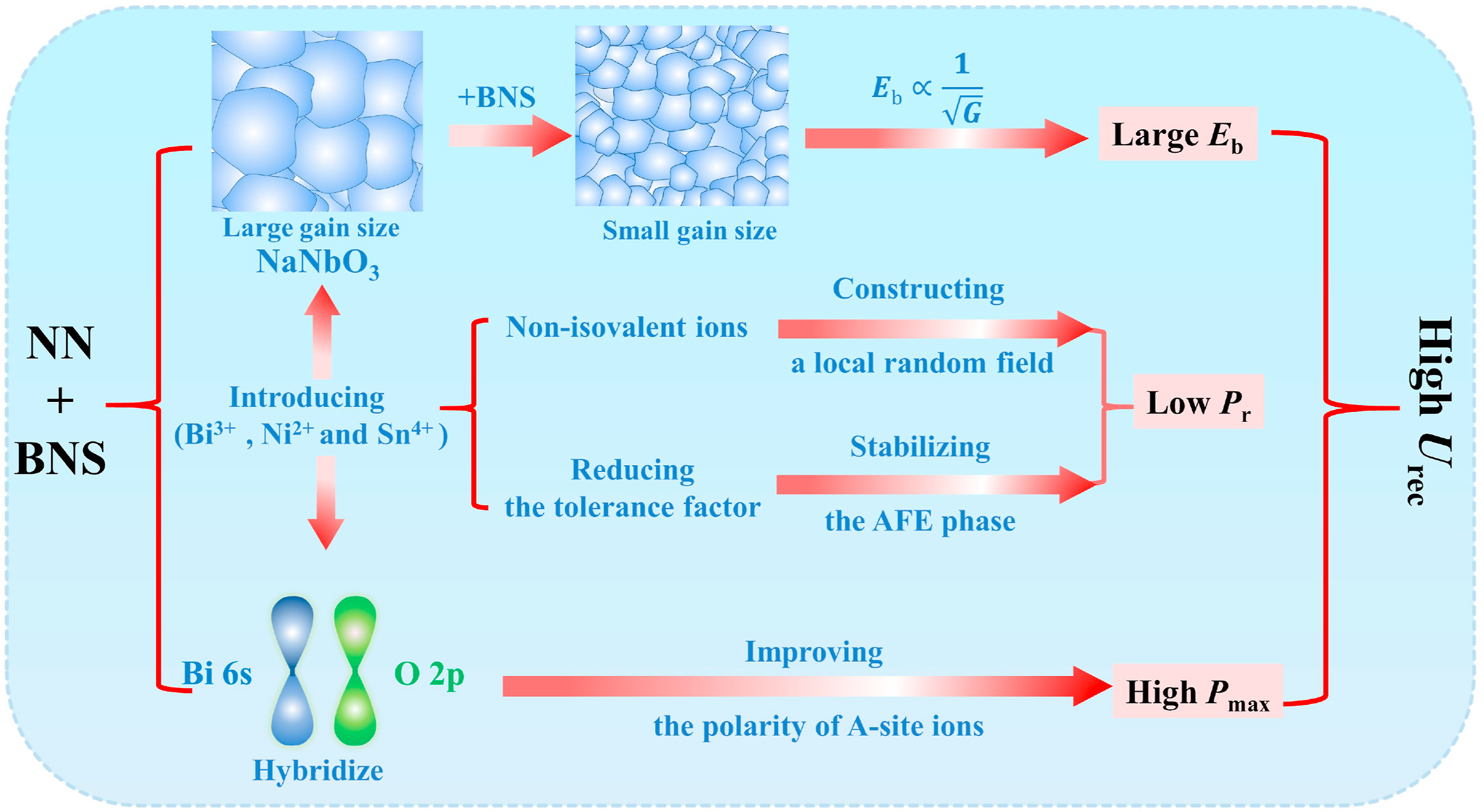
Fig. 1. Schematic diagram of methods applied to achieve a high recoverable energy storage density (Urec).
To test the proposed methods, a novel series of NN-based ceramic systems, xBNS ceramics, was designed and synthesized via enhancing antiferroelectricity and constructing a local random field simultaneously.
2. Experimental procedures
The experimental procedure for the synthesis of (1-x)NaNbO3-xBi(Ni1/2Sn1/2)O3 [briefly as xBNS, x = 0.05, 0.10, 0.15, 0.20, and 0.22] ceramics is described in the supporting material.
3. Results and discussion
3.1. Phase structure and grain size
Fig. 2(a) provides the X-ray diffraction (XRD) patterns of xBNS ceramics at RT with enlargements of the diffraction peaks at (110) and (200). Predictably, the phase structure changed significantly after the addition of BNS. The composition when x = 0.05 exhibited an orthorhombic (O) perovskite structure with the several almost indiscernible AFE peaks [red arrows in Fig. 2(a)]. When x = 0.1, the O phase transformed into the tetragonal (T) phase. The phase structures for 0.10BNS ceramics can be discerned from the Rietveld refinement [Fig. 2(b)]. The small reliability factor [inset of Fig. 2(b)] indicates that these results are reliable. With a further increase in BNS content (0.15 ≤ x ≤ 0.22), the splitting (200) diffraction peaks at 2θ∼46° present low left and high right, and the diffraction peak intensity ratio is 1:2, indicating that the ceramics mainly present a tetragonal structure. In addition, the phase structure of xBNS ceramics gradually transformed into a weakly polar phase with the increasing x, as evidenced by the Raman spectra and ferroelectric data discussed later in this article. In other words, ceramics have a tendency to transform to a pseudo cubic phase, which can also be verified by the gradually merged (200) diffraction peaks. An impurity phase Sn2Nb2O7 (PDF-#: 23–0593) can be observed in x ≥ 0.15, and may have been caused by the volatilization Bi and Na, which have a low melting point. In addition, the diffraction peaks at (110) and (200) gradually moved toward lower angles, implying an enlarged unit cell volume in the xBNS ceramics [31]. Fig. 2(c) presents the representative SEM images and grain size distributions of xBNS ceramics. The average grain size first decreased and then increased as the x value increased [inset of Fig. 2(c)]. The decrease in grain size at low BNS contents was attributed to higher lattice strain energy, while the increase at high BNS contents was ascribed to the absence of Na and Bi [32]. In summary, a relatively small average grain size, a uniform element distribution [see Fig. S2] and a dense microstructure were achieved in 0.10BNS ceramics, which is conducive to acquire a strong Eb.
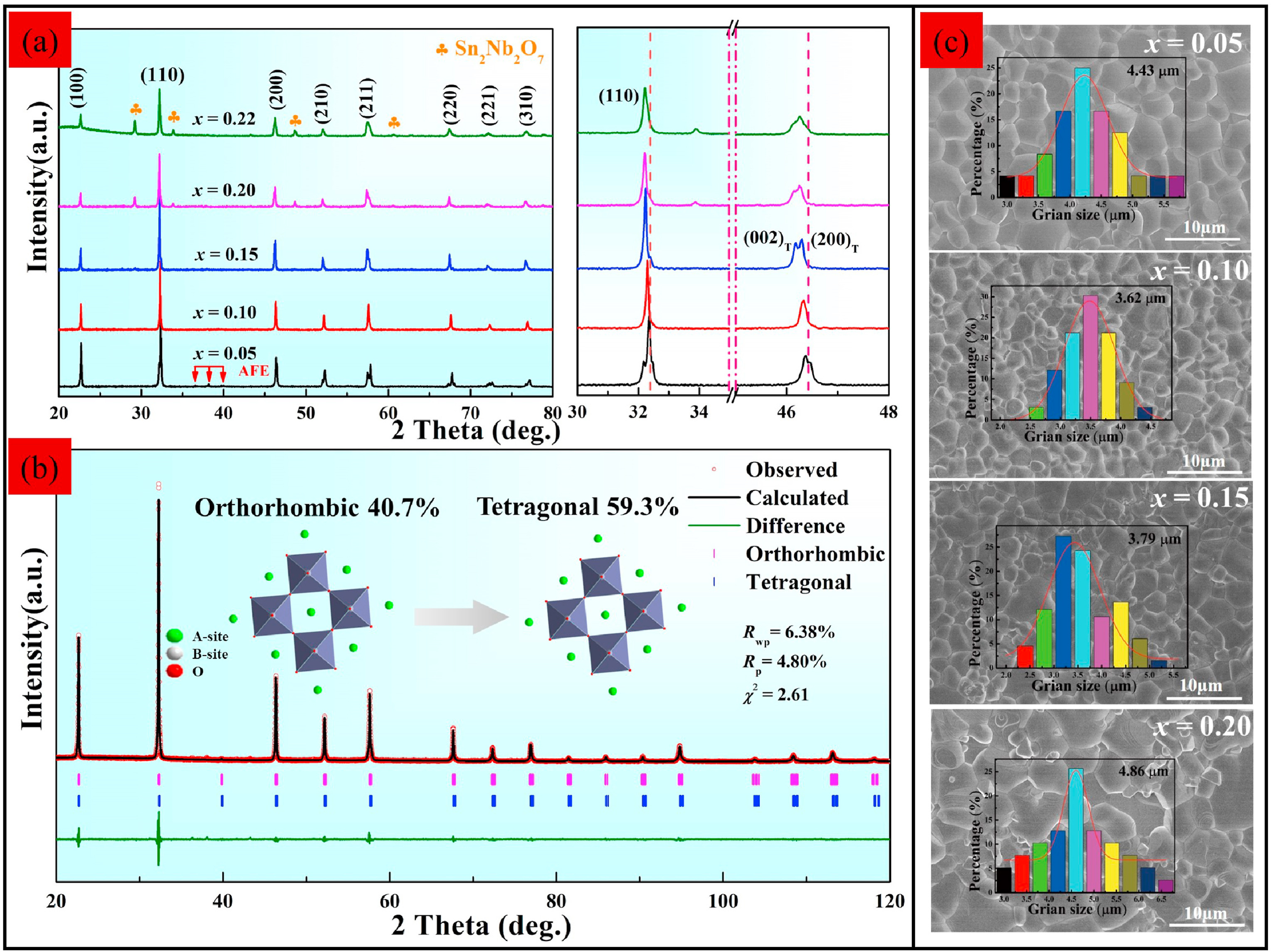
Fig. 2. (a) XRD patterns of xBNS ceramics. (b) Representative SEM images for xBNS ceramics. (c) Rietveld refinement for 0.10BNS at RT.
To better characterize the evolution of local structures, Raman spectroscopy was performed at RT (Fig. 3). Predictably, the number of Raman spectra peaks is significantly reduced once x increased above 0.10, which is consistent with the transition from an O to a T phase, as suggested by the XRD results. Fig. 3(b) displays the spectral deconvolution of all samples using the Gaussian function. The fitted curve (the red line) coincides with the measured data (the black line) and has excellent correlation (R² > 0.9995). The peak positions and the intensities for the different ceramics are presented in Fig. 3(c) and (d). All peaks for the B-O band and the BO6 octahedra region softened in intensity and broadened as the BNS content increased. This was ascribed to an increase in structural disorder and a decrease in the unit cell polarity [33]. Thus, the relaxor characteristics of the system were enhanced by increasing BNS content. These structural changes are conducive to the reduction of the ferroelectric phase in NN ceramics, thereby further optimizing energy storage.
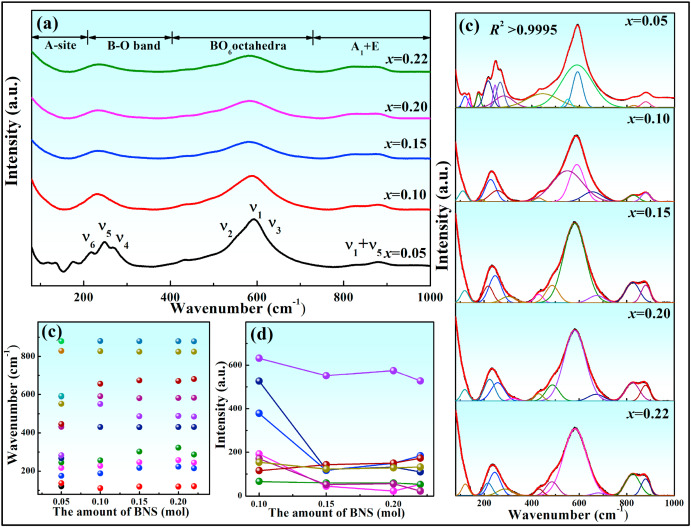
Fig. 3. (a) Raman spectra of xBNS ceramics. (b) Deconvolution of the spectra into Gaussian-Lorentzian-shaped peaks. (c) Variations in peak frequency for different BNS contents. (d) Variations in peak intensity for different BNS contents.
3.2. Dielectric properties
Fig. 4(a) illustrates the temperature dependence of the dielectric properties of xBNS ceramics at 10 kHz. Abnormal dielectric peaks at approximately 0 °C were observed in this system and can be attributed to ice interference at low temperatures [34]. To verify this, a dielectric temperature spectrum test was performed for the 0.1BNS ceramic under vacuum conditions. As seen in the inset of Fig. 4(c), the abnormal dielectric peaks disappeared. Compared to other compositions, a sharp phase transition peak was observed for the 0.05BNS ceramics at approximately 189.7 °C, which may be attributed to the transition from the O phase to paraelectric phase [35]. With the increase in BNS content, the temperature at which the ε was maximized (Tm) decreased [Fig. 4(b)]. The reduced Tm was attributed to the weaker bonding in the NbO6 octahedral network [24]. Broader and less intense dielectric peaks [Fig. 4(c)], which were attributed to the introduction of heterovalent (Bi3+, Ni2+, and Sn4+) ions, are associated with weak relaxor behavior. ε first rised and then decreased with the increasing BNS content [Fig. 4(b)]. The increase in ε at low BNS contents may have been due to the dominant presence of Bi3+ ions at A-sites. ε decreased with the introduction of low-polarization ions (Ni2+, Sn4+) at B-sites, because the polarity of the A-sites was unable to compensate for the loss of the polarity at the B-sites [36]. Fig. 4(d) presents the frequency dependence of ε and dielectric loss (tanδ) on BNS content at RT. ε exhibited a slight decrease above 100 kHz while tanδ exhibited a slight increase, which was ascribed to the dielectric relaxor characteristics of the 0.10BNS ceramics. In addition, all samples, especially the 0.10BNS and 0.15BNS ceramics, displayed ultralow tanδ values (<0.01) in the test frequency range, as well as stable ε and tanδ values, which is conducive to good ESP.
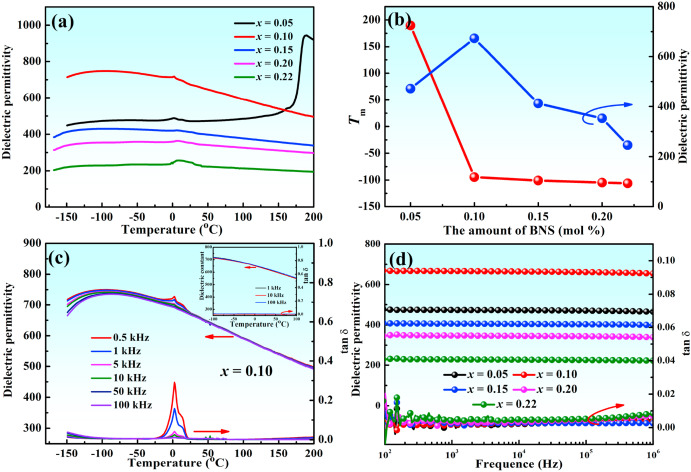
Fig. 4. (a) Temperature dependences of the dielectric constant of xBNS ceramics at 10 kHz. (b) Variation in Tm and εr values of xBNS ceramics vary with BNS content (x). (c) Temperature dependences of the dielectric constant and dielectric loss of 0.10BNS ceramics. (d) Frequency dependence of the dielectric constant and dielectric loss for xBNS ceramics.
3.3. Conduction
To determine the conductive mechanism of xBNS ceramics, impedance spectroscopy was employed (Fig. 5). Predictably, all the as-prepared samples presented a single semicircle on the compound impedance spectroscopy images, indicating that a single relaxor mechanism was dominant in this system [37]. To further estimate the relaxor activation energy (Ea) of the system, we employed a series of equivalent random quantum circuits (RQC) to fit the impedance data, as exhibited in Fig. 5(f). The corresponding conductivity (σB) and Ea were estimated via the following formula [38]: σB=hS×R">σB=h/S×R, σB=σ0exp(−EakBT)">σB=σ0exp(−Ea/kBT), where kB represents the Boltzmann constant, h the thickness, S the tested silver area and R the resistance. The calculated Ea values of the xBNS solid solutions were 1.46, 1.30, 1.21, 1.03, and 1.50 eV for x = 0.05, 0.10, 0.15, 0.20, and 0.22, respectively [Fig. 5(g) and (h)]. These values are indicative of the oxygen vacancy mechanism (0.5–2 eV). A high Ea in xBNS ceramics with a high barrier for the transition of oxygen vacancies may restrict the motion of charge carriers [39], thereby resulting in a high Eb value. Although the 0.05BNS and 0.22BNS ceramics exhibited higher Ea values, their Eb values were lower than those of 0.10BNS ceramics, owing to a large dielectric loss (indicating they are prone to thermal breakdown) and an undesirable microstructure (larger grain size, the existence of second phase, etc.). To sum up, a smaller grain size, more uniform element distribution, denser microstructure and relatively higher Ea were obtained in 0.10BNS ceramics, resulting in improved Eb values.
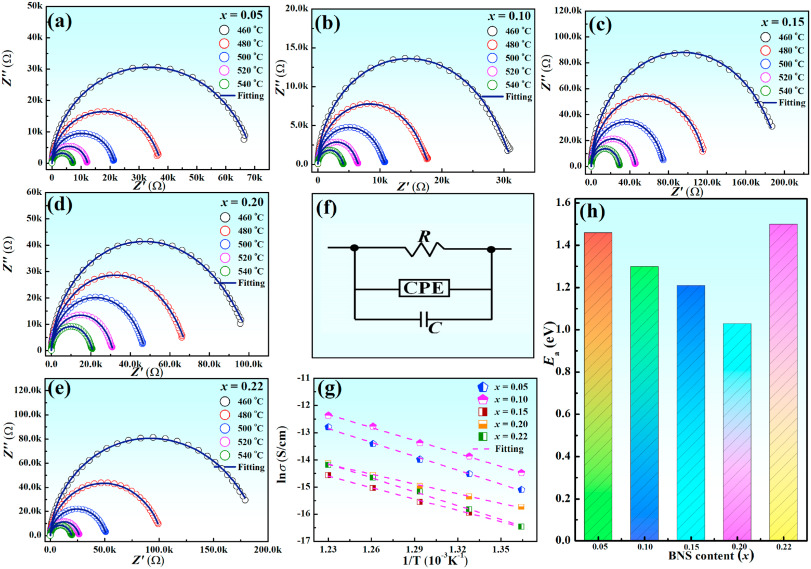
Fig. 5. (a)–(e) Complex impedance for xBNS ceramics at various temperatures. (f) Equivalent circuits fitting impedance data. (g) Arrhenius plots of conductivity vs with temperature. (h) Variation of Ea with BNS content.
3.4. Energy storage properties
To investigate the energy storage properties of xBNS ceramics, the Eb values of all the samples were summarized in Fig. S3 using a Weibull distribution. The calculated Eb values of the xBNS solid solutions were 443, 633, 577, 531 and 488 kV/cm for x = 0.05, 0.10, 0.15, 0.20 and 0.22, respectively. These optimal Eb values are beneficial for ESP. To further clarify the ESP of xBNS ceramics, the unipolar P-E loops of xBNS ceramics in various electric fields are presented in Fig. 6 (a)-(e). When a small amount of BNS was added (∼5%), the as-prepared samples displayed typical AFE characteristics [Fig. 6(a)], indicating that the addition of BNS enhanced the stability of the AFE phase in NN-based ceramics. Similar phenomena were observed in other low-doped NN-based system [35]. Meanwhile, the polarization increased significantly for 0.05BNS ceramics as the electric field strength increased from 230 to 240 kV/cm. Generally, the polarization directions of adjacent dipoles are opposite in AFE ceramics, and the dipoles can be reoriented only when a large enough electric field is applied [35]. At an electric field strength of 230 kV/cm, most of the AFE domains were transformed into FE domains due to the local random field generated by the introduction of non-isovalent ions, which is extremely conducive to enhance polarization [40]. This can be verified by the relationship between energy storage density and electric field in Fig. 6(g). However, due to the limit on the existence of the FE phase, their Pr values were large, resulting in low η. As x increased from 0.05 to 0.22, the P-E loops displaying FE characteristics gradually transformed into slender P-E loops with AFE characteristics. This change was due to enhanced antiferroelectricity and dielectric relaxor behavior, which resulted in a rapid polarization response and significantly increased the phase transition field. The Urec and η values of xBNS ceramics at different electric field strengths are displayed in Fig. 6(f) and (g). The Urec and η values of xBNS ceramics for x = 0.05, 0.10, 0.15, 0.20, and 0.22 measured at Eb are 1.38 J/cm3 and 24.90%, 5.00 J/cm3 and 67.80%, 3.80 J/cm3 and 80.70%, 2.98 J/cm3 and 78.30%, 2.17 J/cm3 and 80.20%, respectively. The Urec and η values of the pure NN ceramics at Eb = 125 kV/cm were 0.14 J/cm3 and 16.45%, respectively (Fig. S4). This significantly enhanced energy storage performance was attributed to the enhanced antiferroelectricity and the formation of a local random field. Fig. 6(h) compares the energy storage densities of various lead-free dielectric ceramic systems [11,17,18,21,24,30,35,36,[41], [42], [43], [44], [45], [46], [47], [48], [49]]. Consequently, one can conclude that xBNS ceramics have potential energy storage applications.
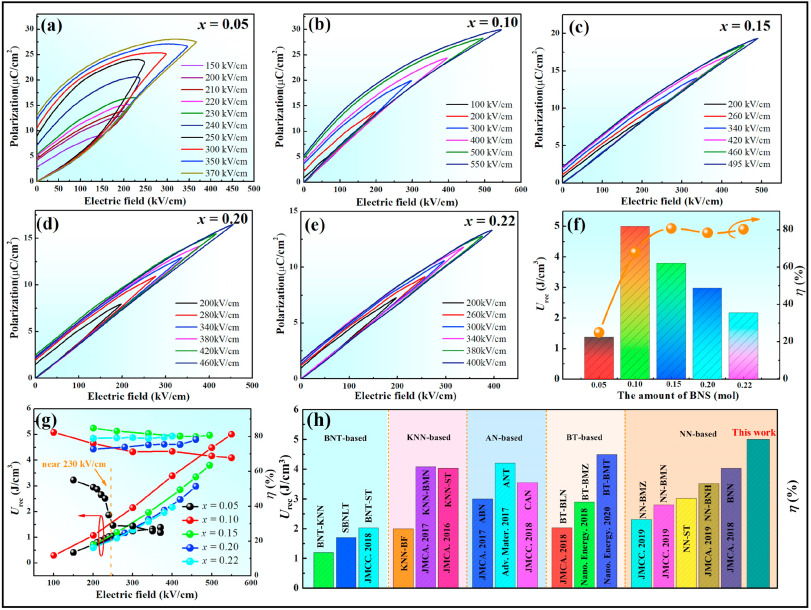
Fig. 6. (a)–(e) Unipolar P-E loops of xBNS ceramics at different electric field strengths. (f) Energy storage properties of xBNS ceramics at different Eb strengths. (g) Energy storage properties of xBNS ceramics at different electric field strengths. (h) A comparison of Urec and η values of 0.10BNS ceramics with those of other reported lead-free ceramics.
The energy storage performance of the 0.10BNS ceramics at different temperatures, frequencies, and fatigue stabilities were studied at Eb = 200 kV/cm (Fig. 7). With respect to temperature stability, as the temperature increased, Urec decreased. However, the variation in Urec was controlled within 11.4% in a temperature range of 20–120 °C. η first increased first and then decreased. The increase in η was due to a decrease in dielectric loss, while the additional increase at high temperatures was attributed to heat accumulation. Similarly, 0.10BNS ceramics exhibited excellent frequency and fatigue stability (variation of Urec ≤ 1.3% in 1–100 Hz and variation of Urec ≤ 6.4% after 104 cycles) (Fig. 7). This decent energy storage stability may be attributed to the compact microstructure and boosting relaxor properties [32].
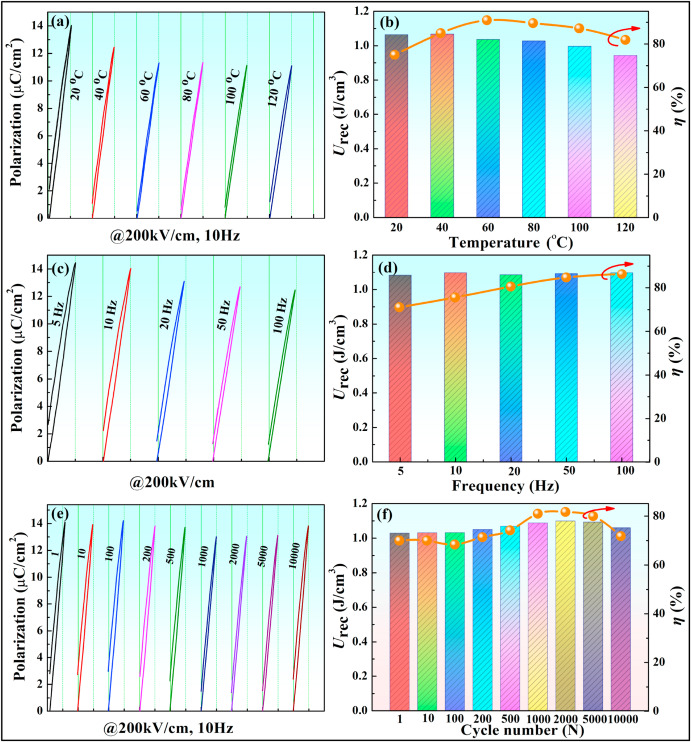
Fig. 7. Energy storage properties of 0.10BNS ceramics at various temperatures, frequencies, and cycles.
3.5. Discharge characteristics
Generally, a strong electric field and high polarization are also conducive to excellent charge and discharge characteristics. Hence, it was necessary to evaluate the discharge characteristics of 0.10BNS ceramics. The underdamped current waveforms of 0.10BNS ceramics are represented in Fig. 8(a), and their corresponding maximum current (Imax), current density (CD=Imax/S), and power density (PD = EImax/2S), where S represents cross-sectional area, are shown in Fig. 8(b). These parameters reached their maximum values, which were 71 A, 1005 A/cm2, and 100.5 W M/cm3, respectively, at Eb = 200 kV/cm [Fig. 8(b)]. The strength of the applied electric field resulting in high charge and discharge rates was significantly lower than the Eb strengths measured by the P-E loops, and was attributed to the difference in timescale between the two measurements. The high charge-discharge rates occurred on the nanosecond or microsecond scale, while the P-E loop measurements were on the millisecond scale [50]. Fig. 8(c) presents the underdamped current waveforms of 0.1BNS ceramics measured at 20–180 °C, and the corresponding Imax, CD and PD values are displayed in Fig. 8(d). The variations of these parameters were less than 11.2% in the temperature range of 20–180 °C, indicating that BNS ceramics have the potential to be used in pulsed power systems in high-temperature applications.Wdis=R∫i2(t)dt/V">Wdis=R∫i²(t)dt/V, where V is the sample volume and R (210 Ω) represents load resistance. The overdamped discharge curves and the dependence of discharge time (t0.9) on Wdis at different electric field strengths are presented Fig. 8(e) and (f) for 0.10BNS ceramics. The maximum current gradually increased with increasing the electric field strength, and Wdis increased from 0.01 to 1.11 J/cm3 [Fig. 8(g)]. In addition, an ultrashort and stable t0.9 of approximately 43.6 ns was achieved for 0.10BNS ceramics at various electric field strengths [Fig. 8(e)]. Fig. 8(h) and (i) exhibit the overdamped discharge current curves, as well as Wdis, and t0.9 at different temperatures. The current density peaked at approximately 14 A/cm2 for various temperatures, whereas Wdis dropped slightly (variation of Wdis <15% in the temperature range of 20–140 °C). The reduction in Wdis was attributed to the decrease in polarization when the temperature rose. Analogous phenomena can be found in other systems [51]. In addition, the t0.9 gradually decreased from 44.2 to 35.8 ns as the temperature rose from 20 °C to 140 °C [Fig. 8(i)]. The stable Wdis and ultrashort t0.9 are extremely favorable for energy storage devices that are required to operate in a broad temperature range.
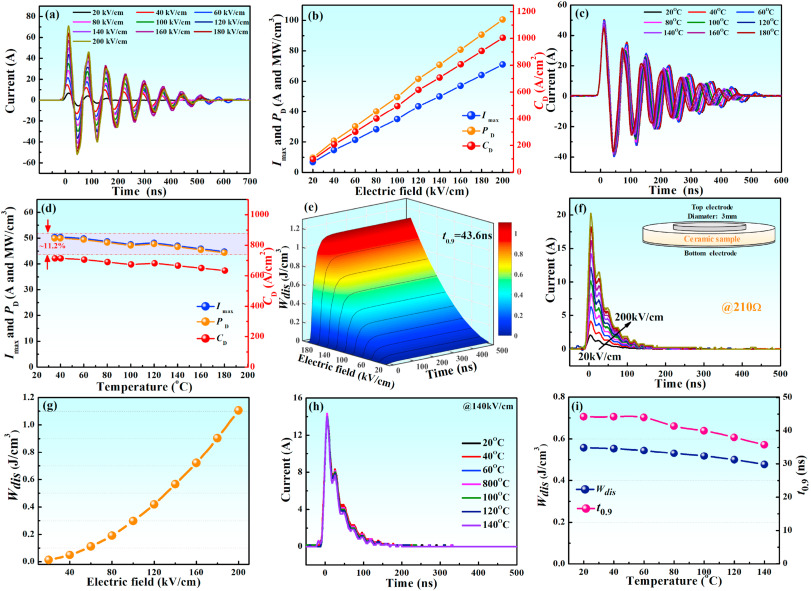
Fig. 8. (a)–(d) Underdamped discharge characteristics of 0.10BNS ceramics under different electric field strengths and temperatures. (e)–(f) Overdamped discharge characteristics of 0.10BNS ceramics at different electric field strengths and temperatures.
3.6. Overall performance
Fig. 9(a) compares the Urec and PD of 0.10BNS ceramics with other lead-free systems [4,35,40,48,[51], [52], [53], [54], [55]]. Fig. 9(a) demonstrates that the overall performance of 0.10BNS ceramics was better than that of the previously reported ceramics. Moreover, in contrast to other pulsed power systems [Fig. 9(b)], 0.10BNS ceramics presented a stable power density in a wide temperature range (20–180 °C). These results indicate that 0.10BNS ceramics are a potential candidate for applications in high-temperature pulsed power systems.
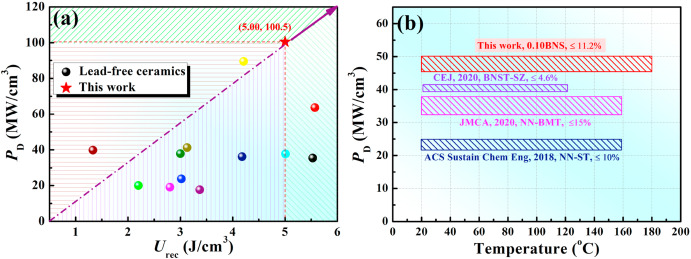
Fig. 9. (a) Wrec and PD values of 0.10BNS ceramics compared with values for other lead-free ceramics. (b) Pulse power stability of 0.10BNS ceramics compared with other lead-free ceramics.
In summary, an ultrahigh Utotal of 7.35 J/cm3, a Urec of 5 J/cm3 at a high Eb strength of 550 kV/cm, along with excellent stability (variation of Urec ≤ 11.4% over 20–120 °C, Urec ≤ 1.3% in 1–100 Hz, and Urec ≤ 6.4% after 104 cycles) were realized in 0.10BNS ceramics. More importantly, an extremely high PD of 100.5 MW/cm3, ultrashort t0.9 of 43.6 ns, and a relatively high pulse discharge energy density (Wdis) of 1.11 J/cm3 were simultaneously realized in 0.10BNS ceramics. These excellent ESP indicate that 0.10BNS ceramics achieved the desired performance in the study.
4. Conclusions
In summary, xBNS ceramics were successfully designed and fabricated as solid-state solutions. An ultrahigh recoverable energy density of 5.00 J/cm3) and a power density of 100.5 W M/cm3 were simultaneously realized in 0.10BNS ceramics via enhanced antiferroelectricity and the development of a local random field. In addition, excellent temperature stability (in the range of 20–120 °C), frequency stability (in the range of 1–100 Hz), and fatigue stability (cycle range of 1−104 cycles) were obtained. Moreover, the 0.10BNS ceramics manifested an ultrashort discharge time (t0.9 = 43.6 ns). These results indicate that this work can guide research into lead-free pulsed power capacitors in the future.
References: omitted
Declaration: This article is provided by CERADIR™ users or obtained from Internet, the content does not represent the position of CERADIR™. We are not responsible for the authenticity/accuracy of the article, especially the effects of the products concerned. This article is for study only, it does not constitute any investment or application advice. For reprinting, please contact the original author. If it involves the copyright and/or other issues, please contact us and we will deal with it asap! CERADIR™ has the interpretation of this declaration.